Foundationalism concerns philosophical theories of knowledge resting upon non-inferential justified belief, or some secure foundation of certainty such as a conclusion inferred from a basis of sound premises.
Here I shall present and defend Modest Foundationalism (MF thereafter) over its competing version in foundationalism, Cartesian Foundationalism (or Strong Foundationalism, CF thereafter), concerning epistemic justification. In general, MF is a response to the objections that CF faces. In the end, this essay will, hopefully, make it clear that MF is better than CF in conducing to proper epistemic justification.
MF's Stand
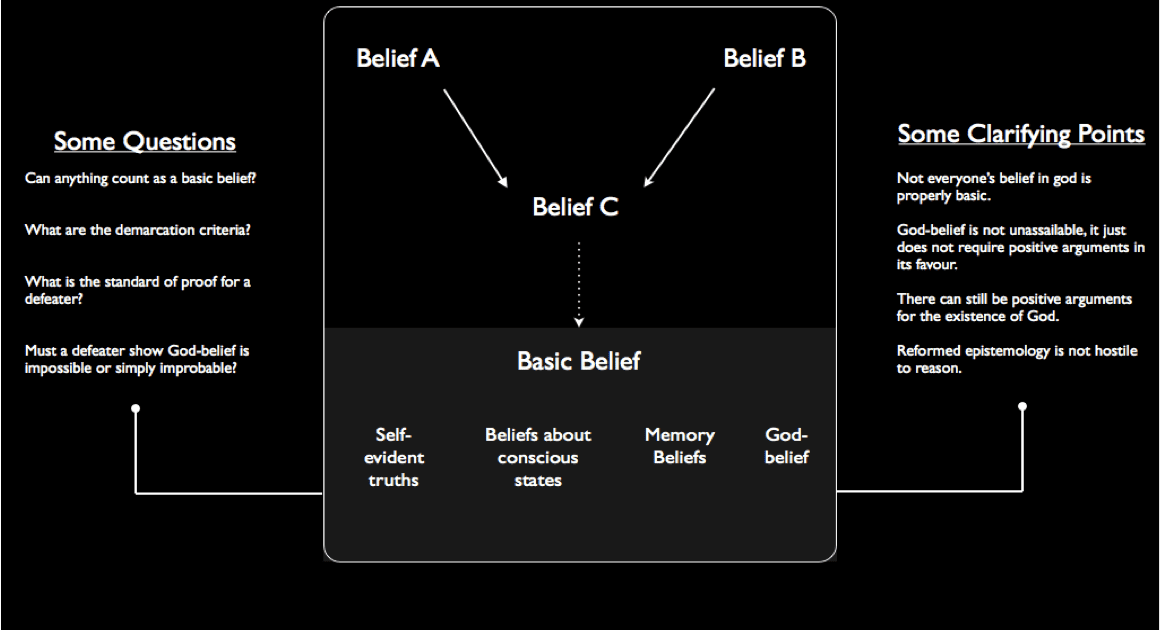
i. Spontaneously formed beliefs can be immediately justified. Typically, these include simple necessary beliefs about the external world (for example, a belief that there is a chair to one’s right) and beliefs about one’s own mental states (for example, a belief that one has a headache right now).
ii. A spontaneously formed belief is immediately justified provided it is a proper response to one’s experiences, and provided it is not defeated by other evidence that one has.
iii. All other justified beliefs are justified because they follow from one’s immediately justified beliefs via deductive inference, inductive inference, or inference to the best explanation.
More specifically, MF’s thesis about the immediate justification of Beliefs formed via perceptions contends that:
Whenever you have an experience as of P’s being the case, you thereby have immediate prima facie justification for believing P.
Prima facie justification is justification that can be defeated by other evidence that one possesses. Examples of defeaters for prima facie justifications in believing P via perception could include:
• Stronger evidence in favour of ¬P.
• Evidence that P’s truth in these circumstances is not ascertainable by perception.
• Evidence that your senses are malfunctioning.
MF does not hold that beliefs formed spontaneously via perception are prima facie justified because perceptual experiences tend to be reliable.
CF's Stand
i. Beliefs about one’s own inner state of mind (e.g., appearance beliefs) and beliefs about simple necessary truths (e.g., beliefs about elementary truths of logic and mathematics) can be immediately justified.
ii. Immediately justified beliefs are justified because we are infallible about such matters.
iii. All other justified beliefs are justified because they can be deduced from one’s immediately justified beliefs.
Where's the Prob?
CF states that beliefs about one’s own inner state of mind is infallible, but actually they are fallible. Arguably, the following is an example in which “I believe that I feel something hot but am wrong”:
The Initiation Trick: As part of an initiation ritual for some secret club, I am blindfolded and told that I will be branded with a red-hot poker that I can hear (and smell) being heated for that purpose. When a piece of ice is then pushed into my bare stomach, I scream, for I mistakenly believe myself to be feeling a sensation of intense heat.
This problem is successfully tackled by MF, as mentioned in the elaboration part, the immediate prima facie perceptual justification in MF is open to be defeated by other evidence that one possesses. In this case, knowing that the poker is actually a piece of ice after the initiation trick is robust evidence that my senses are malfunctioning. So, basic beliefs of MF are open to proper amelioration, leading to more comprehensive epistemic justifications, but those of CF are not.
Another more salient problem with CF that MF explains well has to do with inferential justification. CF requires that all non-basic justified beliefs are justified because they can be deduced from one’s immediately justified beliefs, this is too high a standard for justification. Non-basic beliefs can be justified even if they can’t be deduced from basic ones, as MF states, “through deductive inference, inductive inference, or inference to the best explanation.”
Consider the following case: There is a man A, who is walking down a street. In the distance, he sees another man B running out of a house with some objects, and as he is running away, he maintains a panicked look on his face. Additionally, when A walks by the house in question, he sees a window that has a gigantic hole with glass shards everywhere. So A formed immediately justified basic beliefs through visual perception that the window is broken and B is frantically fleeing away from the house with some objects. Let’s call these basic beliefs evidence set “E”, from E, A deduces the non-basic justified belief Q that B has escaped from that house. Then, by applying inferential justification, A forms another justified non-basic belief P that B had stolen those objects from that house. In this case, P is justified because it arises from inference to the best explanation through justified non-basic belief Q, instead of being directly deduced from E.
Considering that MF allows for inductive justification, there might be possible objections. For example, David Hume suggests that our inductive inference process can be described as below:
P: All observed instances of A have been B.
C: The next instance of A will be B.
He argues that such a pattern presupposes the Uniformity Principle, i.e., we are presuming that the future will be like the past that we have observed, thus ignoring possible deviations. And the process repeats itself, leading to circular argument.
Notwithstanding, the objection above leaves untouched the question of how we distinguish between cases where we extrapolate a regularity legitimately, regarding it as a law, and cases where we do not.
Suppose we define a predicate “grue” in the following way. An object is “grue” when it is green if observed before time t and blue otherwise. And we observe a bunch of grue emeralds before time t. We could say that all the observed emeralds are green. Using a simple enumerative inductive schema, we could infer that all emeralds are green. But using the same inductive schema, we could also infer from the result that all observed emeralds are grue, that all emeralds are grue. In the first case, we expect an emerald observed after time t to be green, whereas in the second, we expect it to be blue. Thus, the two predictions are incompatible.
From the case above, we can see that there is not one general Uniformity Principle that all probable arguments rely upon, rather each inductive inference presupposes some more specific empirical presupposition. The nature of this objection is thus transformed from circular argument to an infinite regress problem of inductive presuppositions, which is already solved by foundationalism itself.
Comments | NOTHING